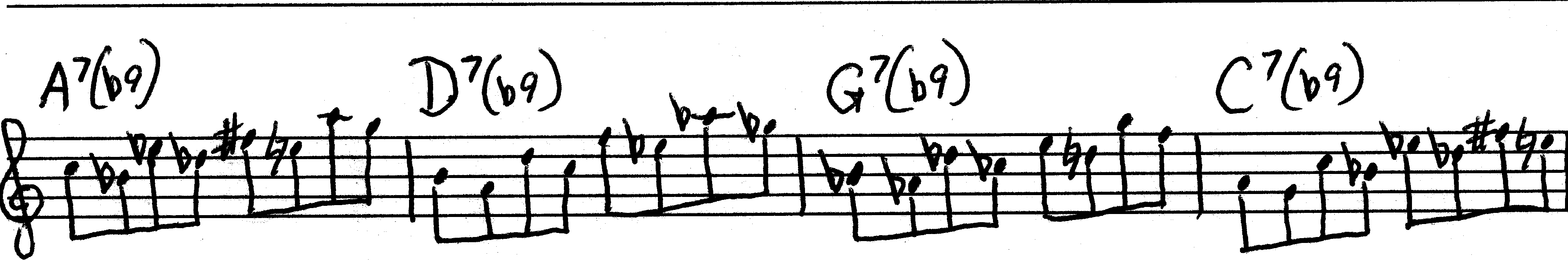The following pattern is nothing new. If you’ve worked at all on the diminished scale, you’ve doubtless encountered it. But have you worked it out in descending half-steps until it lies smoothly under your fingers from the top to the bottom of your horn? Because that root movement downward by minor seconds is what makes it, or any diminished pattern, particularly useful.
Let’s take a look at why this is. First, here’s the lick:
Note that while each measure brings the diminished scale pattern progressively downward by a half-step, the corresponding chord progression is moving around the circle of fifths. Why?
Remember tritone substitution?
The diminished scale functions perfectly as a scale of choice for V7b9 chords and their tritone substitutes because of its symmetrical nature. Due to its structure, every diminished scale will accommodate not just one, but four possible V7b9 chords.
For instance, a half-step/whole-step diminished scale beginning on the note A will obviously work with an A7b9 chord. But it will also work equally well with C7b9, Eb7b9, and F#7b9.
Note that the A7b9 and Eb7b9 have roots a tritone apart, as do the C7b9 and F#7b9. In other words, tritone substitution is hardwired into the diminished scale.
Herein lies the magic of diminished scales descending by half-steps.
Look closely at the above diminished pattern and you’ll note that root movement downward by a half-step gives you the same scales that you’d arrive at with the circle of fifths.
For instance, in the first bar, the diminished pattern starting on the note C repeats itself sequentially a half-step down in the following measure, starting on the note B.
But what scale would you have arrived at if, instead of dropping a by a minor second, you had dropped by a perfect fifth to the note F, as you would do if you were moving around the circle of fifths? Guess what? You’d have the same scale–i.e. B, C, D, Eb, F, Gb, Ab, A. You’d just be starting on a different note of that scale.
Now, if you’re thinking…
…you’ll realize that because every diminished scale contains four possible roots, you can also achieve the results I’ve just described by moving upward by major seconds, another easy root movement.
You can also move down by a major third or a perfect fifth, but it’s easier to focus on the two root movements that are close at hand.
In summary
When woodshedding a diminished scale lick, it particularly behooves you to get it laying smoothly under your fingers in root movements of descending half-steps and ascending whole steps.
And that, Grasshopper, is that. The rest is up to you.